De Sitter space
|
Read other articles:

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Float – berita · surat kabar · buku · cendekiawan · JSTOR FloatAsalJakarta, IndonesiaGenrePop, Rock, FolkTahun aktif2004 - sekarangLabelFloat ProjectAnggotaHotma Roni Simamora David Qlintang Binsar Tobing T…

County Kingfisher, OklahomaKantor pos di kota Kingfisher (2014)Map of Oklahoma highlighting County KingfisherLokasi di negara bagian OklahomaLokasi negara bagian Oklahoma di Amerika SerikatDidirikan2 Mei 1890SeatKingfisherKota terbesarKingfisherWilayah • Keseluruhan906 sq mi (2.347 km2) • Daratan898 sq mi (2.326 km2) • Perairan7,9 sq mi (20 km2), 0,9%Populasi (est.) • (2019)15.765 • …

Vergeltungswaffe 2V-2, Aggregat-4, A-4Un missile V2 al museo di PeenemündeDescrizioneImpiegorappresaglia Sistema di guidaradio Impostazione1936 In servizio1944 Ritiro dal servizio1945 Utilizzatore principaleGermania nazista Esemplari4 000 Peso e dimensioniPeso13500 kg Altezza14 m Diametro1,65 m PrestazioniGittata320-360 km Velocità massima5200 km/h EsplosivoTritolo e Nitrato di Ammonio, 800 kg voci di missili presenti su Wikipedia Il missi…

Artikel ini bukan mengenai Universitas Semarang.Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Universitas Negeri Semarang – berita · surat kabar · buku · cendekiawan · JSTORartikel ini perlu dirapikan agar memenuhi standar Wikipedia. Tidak ada alasan y…

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目需要补充更多来源。 (2018年3月17日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:羅生門 (電影) — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 此�…

Seine-Maritime Région Haute-Normandie Préfecture Rouen Président Didier Marie Arrondissement 3 Kanton 69 Kotamadya 745 Wilayah 6.278 km² Penduduk (1999) - Total - Kepadatan 1.239.138 hab. 197 hab./km² Seine-Maritime di Prancis. Seine-Maritime ialah sebuah departemen di Normandia, Prancis. Lihat pula Seine lbsDepartemen di Prancis 01 Ain 02 Aisne 03 Allier 04 Alpes-de-Haute-Provence 05 Hautes-Alpes 06 Alpes-Maritimes 07 Ardèche 08 Ardennes 09 Ariège 10 Aube 11 Aude 12 Aveyron 13…

Artikel ini memiliki beberapa masalah. Tolong bantu memperbaikinya atau diskusikan masalah-masalah ini di halaman pembicaraannya. (Pelajari bagaimana dan kapan saat yang tepat untuk menghapus templat pesan ini) Artikel ini sebagian besar atau seluruhnya berasal dari satu sumber. Diskusi terkait dapat dibaca pada the halaman pembicaraan. Tolong bantu untuk memperbaiki artikel ini dengan menambahkan rujukan ke sumber lain yang tepercaya. Artikel ini perlu diwikifikasi agar memenuhi standar kualita…

العلاقات الإسبانية الباكستانية إسبانيا باكستان إسبانيا باكستان تعديل مصدري - تعديل العلاقات الإسبانية الباكستانية هي العلاقات الثنائية التي تجمع بين إسبانيا وباكستان.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه …

العلاقات المغربية الكرواتية المغرب كرواتيا المغرب كرواتيا تعديل مصدري - تعديل العلاقات المغربية الكرواتية هي العلاقات الثنائية التي تجمع بين المغرب وكرواتيا.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه المقارنة �…

Names Simplified Chinese: 郭嘉 Traditional Chinese: 郭嘉 Pinyin: Guō Jiā Wade-Giles: Kuo Chia Zi: Fengxiao (奉孝) Guo Jia (Hanzi: 郭嘉; 170 - 207), bernama lengkap Guo Fengxiao (郭奉孝), adalah salah satu ahli strategi Cao Cao pada Zaman Tiga Negara. Pada awalnya ia mengabdi kepada Yuan Shao. Ia kemudian menjadi penasehat Cao Cao setelah direkomendasikan oleh Xun Yu. Selama 11 tahun membantu Cao Cao, kecerdasannya sangat berperan dalam menaklukkan Lu Bu, Yuan Shao, dan pemimpin suku…

Fictional characters Comics character ElvesGrendell the Dark Elf on the cover of The Mighty Thor vol. 1, #377 (March 1987). Art by Walt Simonson.Publication informationPublisherMarvel ComicsFirst appearanceThor #344 (June 1984)Created byVariousIn-story informationPlace of originAsgard There are many fictional Elves appearing in American comic books published by Marvel Comics. The most common of the Elves are the Dark Elves of Svartalfheim and the Light Elves of Alfheim that are based on the elve…

Questa voce sull'argomento calciatori italiani è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Giovanni Lanfritto Nazionalità Italia Calcio Ruolo Difensore Termine carriera 1933 Carriera Squadre di club1 1913-1920 Cremonese27 (1)1920-1922 Brescia31 (1)1923-1924 Cremonese9 (0)1924-1925 Inter15 (4)1925-1930 Canottieri Lecco? (?)19??-1933 Erbese? (?) 1 I due numeri indicano le …
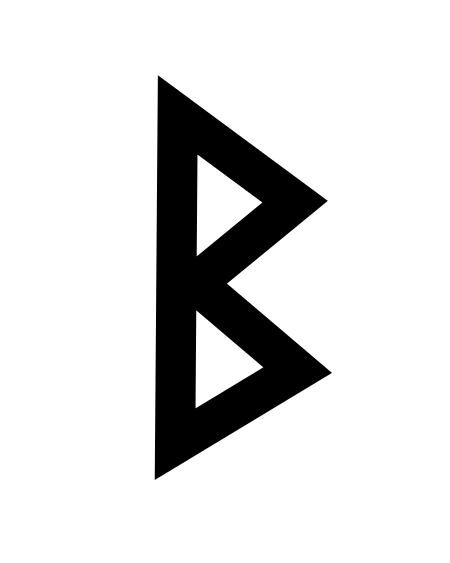
此條目介紹的是拉丁字母中的第2个字母。关于其他用法,请见「B (消歧义)」。 提示:此条目页的主题不是希腊字母Β、西里尔字母В、Б、Ъ、Ь或德语字母ẞ、ß。 BB b(见下)用法書寫系統拉丁字母英文字母ISO基本拉丁字母(英语:ISO basic Latin alphabet)类型全音素文字相关所属語言拉丁语读音方法 [b][p][ɓ](适应变体)Unicode编码U+0042, U+0062字母顺位2数值 2歷史發展…

Gojōme 五城目町KotaprajaBalai Kota Gojōme BenderaEmblemLokasi Gojōme di Prefektur AkitaGojōmeLokasi di JepangKoordinat: 39°56′38″N 140°6′41.9″E / 39.94389°N 140.111639°E / 39.94389; 140.111639Koordinat: 39°56′38″N 140°6′41.9″E / 39.94389°N 140.111639°E / 39.94389; 140.111639Negara JepangWilayahTōhokuPrefektur AkitaDistrikMinamiakitaPemerintahan • WalikotaHikobē WatanabeLuas • Total…

Football match1995 Football League First Division play-off FinalEvent1994–95 Football League First Division Bolton Wanderers Reading 4 3 (a.e.t.)Date29 May 1995VenueWembley Stadium, LondonRefereePeter FoakesAttendance64,107← 1994 1996 → The 1995 Football League First Division play-off Final was an association football match which was played on 29 May 1995 at Wembley Stadium, London, between Bolton Wanderers and Reading. The match was to determine the second and final team to gain p…

Protein-coding gene in the species Homo sapiens POU4F1IdentifiersAliasesPOU4F1, BRN3A, Oct-T1, RDC-1, brn-3A, POU class 4 homeobox 1, ATITHSExternal IDsOMIM: 601632 MGI: 102525 HomoloGene: 21255 GeneCards: POU4F1 Gene location (Human)Chr.Chromosome 13 (human)[1]Band13q31.1Start78,598,362 bp[1]End78,603,552 bp[1]RNA expression patternBgeeHumanMouse (ortholog)Top expressed insecondary oocytespinal gangliatrigeminal gangliontibialis anterior musclepancreatic ductal cellmedul…

Ornamen giok Tionghoa, dengan rancangan naga dan elang, dari akhir zaman Musim Semi dan Musim Gugur (722–482 SM). Awal Musim Gugur, abad ke-13, karya pelukis loyalis Song Qian Xuan. Gambar dedaunan teratai dan capung-capung beterbangan di atasair diyakini adalah sebuah kritikan terselubung terhadap kekuasaan Mongol.[1] Nampan tanah liat yang diukir dengan dua burung berada pada latar belakang bunga-bungaan, lebar 19 cm, abad ke-13 Potret dari Kaisar Dinasti Yuan Kubilai Khan Bagia…

Федеральное агентство по делам Содружества Независимых Государств, соотечественников, проживающих за рубежом, и по международному гуманитарному сотрудничествусокращённо: Россотрудничество Общая информация Страна Россия Юрисдикция Россия Дата создания 6 сентября 20…

坐标:43°11′38″N 71°34′21″W / 43.1938516°N 71.5723953°W / 43.1938516; -71.5723953 此條目需要补充更多来源。 (2017年5月21日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:新罕布什尔州 — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(…

斯洛博丹·米洛舍维奇Слободан МилошевићSlobodan Milošević 南斯拉夫联盟共和国第3任总统任期1997年7月23日—2000年10月7日总理拉多耶·孔蒂奇莫米尔·布拉托维奇前任佐兰·利利奇(英语:Zoran Lilić)继任沃伊斯拉夫·科什图尼察第1任塞尔维亚总统任期1991年1月11日[注]—1997年7月23日总理德拉古京·泽莱诺维奇(英语:Dragutin Zelenović)拉多曼·博若维奇(英语:Radoman Božov…











































